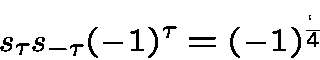Multiplier theorem for $1$-perfect sequences
 

 
Belevitch [19xx]. Let 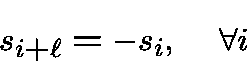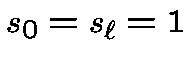$s$ be a $1$-perfect sequence of period $v=2\ell$ satisfying
\begin{displaymath}s_{i+\ell} = -s_{i},\quad\forall i\end{displaymath}
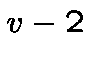excepted $s_0=s_{\ell}=1$. Let us set $s_0=0$ and $s_\ell=0$. We obtain a sequence of type II of weight$v-2$ that satisfies
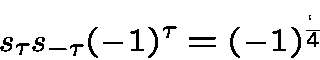
\begin{displaymath}s_\tau s_{-\tau} (-1)^{\tau} = (-1)^{\ell\over 4}\end{displaymath}

 
Assume $0 < \tau <{\ell\over 2}$
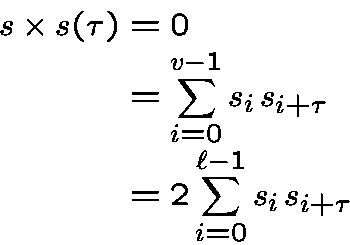
\begin{displaymath}\begin{split}s\times s(\tau) &=0\\&=\sum_{i=0}^{v-1} s_i\......tau}\\&=2 \sum_{i=0}^{\ell-1} s_i\,s_{i+\tau}\\\end{split}\end{displaymath}


In the sum
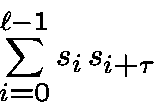
\begin{displaymath}\sum_{i=0}^{\ell-1} s_i\,s_{i+\tau}\end{displaymath}
we have 2 terms equals to $0$ and $\ell-2$ terms equal to $\pm 1$.The product of the non-zero terms is equal to
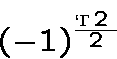
\begin{displaymath}(-1)^{{\ell-2}\over 2}\end{displaymath}
Let us replace $s_{i+\tau}$ by $-s_{i+\tau-\ell}$ when $i+\tau\geq \ell$. For $1\leq i\leq \ell-1$, $s_i$ appears twice except $s_\tau$ and$s_{\ell-\tau}$ which appears only once. Because of the signs the products of all the terms is equal to
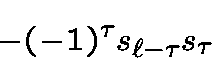
\begin{displaymath}-(-1)^{\tau}s_{\ell-\tau}s_{\tau}\end{displaymath}
Finally,
\begin{displaymath}s_\tau s_{-\tau} (-1)^{\tau} = (-1)^{\ell\over 4}\end{displaymath}